THE LINK BETWEEN SIMPLE HARMONIC MOTION AND WAVES .
If a time-exposure photograph of the bouncing car were taken as it drove by, the headlight would make a wavelike streak, as shown in Figure 1. Similarly, Figure 2 shows an object bouncing on a spring as it leaves a wavelike "trace of its position on a moving strip of paper. Both waves are sine functions. All simple harmonic motion is intimately related to sine and cosine waves.
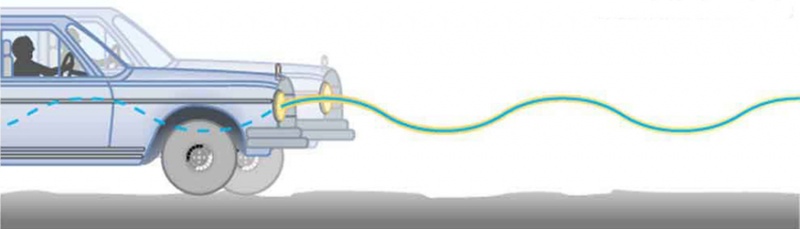
Figure 1 The bouncing car makes a wavelike motion. If the restoring force in the suspension system can be described only by Hooke’s law, then the wave is a sine function. (The wave is the trace produced by the headlight as the car moves to the right.)

Figure 2 The vertical position of an object bouncing on a spring is recorded on a strip of moving paper, leaving a sine wave.
The displacement as a function of time t in any simple harmonic motion—that is, one in which the net restoring force can be described by Hooke’s law, is given by
\(x(t) = X \thinspace \cos \frac{2 \pi}{T},\)
where \(X\) is amplitude. At \(t = 0\), the initial position is \(x_{0} = X\), and the displacement oscillates back and forth with a period \(T\). (When \(t = T\), we get \(x = X\) again because \(\cos \thinspace 2 \pi = 1\).). Furthermore, from this expression for x, the velocity v as a function of time is given by:
\(v(t) = -v_{\mathrm{max}} \thinspace \sin \left ( \frac{2 \pi t}{T} \right ),\)
where \(v _{\mathrm{max}} = 2 \pi X/T = X \sqrt{k/m}\). The object has zero velocity at maximum displacement—for example, \(v = 0\) when \(t = 0\), and at that time \(x = X\). The minus sign in the first equation for \(v (t)\) gives the correct direction for the velocity. Just after the start of the motion, for instance, the velocity is negative because the system is moving back toward the equilibrium point. Finally, we can get an expression for acceleration using Newton’s second law. [Then we have \(x(t)\),\(v(t)\),\(t\), and \(a(t)\), the quantities needed for kinematics and a description of simple harmonic motion.] According to Newton’s second law, the acceleration is \(a = F/m = kx/m\). So, \(a(t)\) is also a cosine function:
\(a(t) = - \frac{kX}{m} \cos \frac{2 \pi t}{T}.\)
Hence, \(a(t)\) is directly proportional to and in the opposite direction to \(x(t)\).
Figure 3 shows the simple harmonic motion of an object on a spring and presents graphs of \(x(t),v(t)\) and \(a(t)\) versus time.

Figure 3 Graphs of \(x(t),v(t)\) and \(a(t)\) versus t for the motion of an object on a spring. The net force on the object can be described by Hooke’s law, and so the object undergoes simple harmonic motion. Note that the initial position has the vertical displacement at its maximum value \(X;v\) is initially zero and then negative as the object moves down; and the initial acceleration is negative, back toward the equilibrium position and becomes zero at that point.
The most important point here is that these equations are mathematically straightforward and are valid for all simple harmonic motion. They are very useful in visualizing waves associated with simple harmonic motion, including visualizing how waves add with one another.
PHET EXPLORATIONS MASSES AND SPRINGS
A realistic mass and spring laboratory. Hang masses from springs and adjust the spring stiffness and damping. You can even slow time. Transport the lab to different planets. A chart shows the kinetic, potential, and thermal energy for each spring.
Click here to view the simulation page